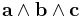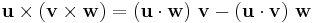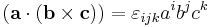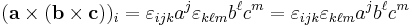Triple product
In mathematics, the triple product is a product of three vectors. The name "triple product" is used for two different products, the scalar-valued scalar triple product and, less often, the vector-valued vector triple product.
Contents |
Scalar triple product
The scalar triple product (also called the mixed or box product) is defined as the dot product of one of the vectors with the cross product of the other two.
Geometric interpretation
Geometrically, the scalar triple product
is the (signed) volume of the parallelepiped defined by the three vectors given.
Properties
The scalar triple product can be evaluated numerically using any one of the following equivalent characterizations:
Switching the two vectors in the cross product negates the triple product, i.e.:
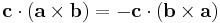 .
.
The parentheses may be omitted without causing ambiguity, since the dot product cannot be evaluated first. If it were, it would leave the cross product of a scalar and a vector, which is not defined.
The scalar triple product can also be understood as the determinant of the 3 × 3 matrix having the three vectors as its rows or columns (the determinant of a transposed matrix is the same as the original); this quantity is invariant under coordinate rotation.
Note that if the scalar triple product is equal to zero, then the three vectors a, b, and c are coplanar, since the "parallelepiped" defined by them would be flat and have no volume.
There is also this property of triple products:
Scalar or pseudoscalar
Although the scalar triple product gives the volume of the parallelepiped, it is the signed volume, the sign depending on the orientation of the frame or the parity of the permutation of the vectors. This means the product is negated if the orientation is reversed, for example by a parity transformation, and so is more properly described as a pseudoscalar if the orientation can change.
This also relates to the handedness of the cross product; the cross product transforms as a pseudovector under parity transformations and so is properly described as a pseudovector. The dot product of two vectors is a scalar but the dot product of a pseudovector and a vector is a pseudoscalar, so the scalar triple product must be pseudoscalar valued.
As an exterior product
In exterior algebra and geometric algebra the exterior product of two vectors is a bivector, while the exterior product of three vectors is a trivector. A bivector is an oriented plane element and a trivector is an oriented volume element, in the same way that a vector is an oriented line element. Given vectors a, b and c, the product
is a trivector with magnitude equal to the scalar triple product, and is the pseudoscalar dual of the triple product. As the exterior product is associative brackets are not needed as it does not matter which of a ∧ b or b ∧ c is calculated first, though the order of the vectors in the product does matter. Geometrically the trivector a ∧ b ∧ c corresponds to the parallelepiped spanned by a, b, and c, with bivectors a ∧ b, b ∧ c and a ∧ c matching the parallelogram faces of the parallelepiped.
Vector triple product
The vector triple product is defined as the cross product of one vector with the cross product of the other two. The following relationships hold:
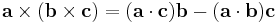
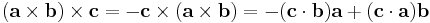 .
.
The first formula is known as triple product expansion, or Lagrange's formula,[1][2] although the latter name is ambiguous (see disambiguation page). Its right hand member is easier to remember by using the mnemonic “BAC minus CAB”, provided one keeps in mind which vectors are dotted together. A proof is provided below.
These formulas are very useful in simplifying vector calculations in physics. A related identity regarding gradients and useful in vector calculus is Lagrange's formula of vector cross-product identity:[3]
This can be also regarded as a special case of the more general Laplace-de Rham operator 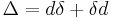 .
.
Proof
The x component of 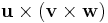 is given by:
is given by:
- uy(vxwy − vywx) − uz(vzwx − vxwz)
or
- vx(uywy + uzwz) − wx(uyvy + uzvz)
By adding and subtracting uxvxwx, this becomes
- vx(uxwx + uywy + uzwz) − wx(uxvx + uyvy + uzvz) =
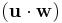 vx −
vx − 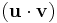 wx
wx
Similarly, the y and z components of 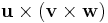 are given by:
are given by:
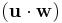 vy −
vy − 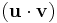 wy
wy
and
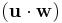 vz −
vz − 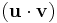 wz
wz
By combining these three components we obtain:
Vector or pseudovector
Where parity transformations need to be considered, so the cross product is treated as a pseudovector, the vector triple product is vector rather than pseudovector valued, as it is the product of a vector a and a pseudovector b × c. This can also be seen from the expansion in terms of the dot product, which consists only of a sum of vectors multiplied by scalars so must be vector valued.
Notation
Using the Levi-Civita symbol, the triple product is
and
which can be simplified by performing a contraction on the Levi-Civita symbols, 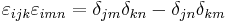 and simplifying the result.
and simplifying the result.
See also
Note
- ^ Joseph Louis Lagrange did not develop the cross product as an algebraic product on vectors, but did use an equivalent form of it in components: see Lagrange, J-L (1773). "Solutions analytiques de quelques problèmes sur les pyramides triangulaires". Oeuvres. vol 3. He may have written a formula similar to the triple product expansion in component form. See also Lagrange's identity and Kiyoshi Itō (1987). Encyclopedic Dictionary of Mathematics. MIT Press. p. 1679. ISBN 0262590204.
- ^ Kiyoshi Itō (1993). "§C: Vector product". Encyclopedic dictionary of mathematics (2nd ed.). MIT Press. p. 1679. ISBN 0262590204. http://books.google.com/books?id=azS2ktxrz3EC&pg=PA1679.
- ^ Pengzhi Lin (2008). Numerical Modelling of Water Waves: An Introduction to Engineers and Scientists. Routledge. p. 13. ISBN 0415415780. http://books.google.com/books?id=x6ALwaliu5YC&pg=PA13.
- ^ J. Heading (1970). Mathematical Methods in Science and Engineering. American Elsevier Publishing Company, Inc. pp. 262–263.
References
- Lass, Harry (1950). Vector and Tensor Analysis. McGraw-Hill Book Company, Inc.. pp. 23–25.
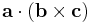
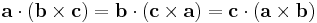
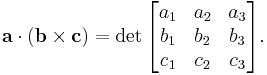
![[\mathbf{a}\cdot(\mathbf{b}\times \mathbf{c})] \mathbf{a}=(\mathbf{a}\times \mathbf{b})\times (\mathbf{a}\times \mathbf{c})](/2012-wikipedia_en_all_nopic_01_2012/I/a9f42fd2108df572cc6da27bdffbce2b.png)